
Wählen Sie eine
oder mehrere Sprachen aus
0,1,0
- Deutsch
- Englisch
- Chinesisch
- Spanisch
Weg-Zeit-Diagramm
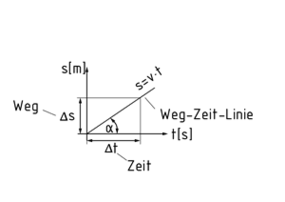
Das Weg-Zeit-Diagramm veranschaulicht den zurückgelegten Weg s eines bewegten Körpers über die Zeit t in grafischer Form. Die Steigung $\left( \frac{ds}{dt} \right)$ des Graphen entspricht dabei der momentanen Geschwindigkeit v des Körpers.
Verläuft der Graph als Gerade, so handelt es sich um eine gleichförmige Bewegung $(v=konst.,a=0)$ . Die Steigung des Graphen ist dann als $\tan \alpha =\frac{\Delta s}{\Delta t}$ berechenbar.
Bei konstanter Beschleunigung $(a=konst.)$ verläuft der Graph des Weg-Zeit-Diagramms in Form einer Parabel.
Path-time diagram
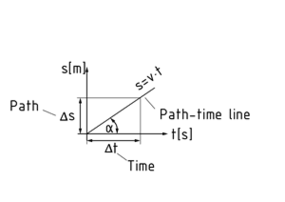
A path-time diagram is a graphical depiction of the path s covered by a moving body over time t. The pitch $\left( \frac{ds}{dt} \right)$ of the line corresponds to the speed v of the body at any one time.
If the graph is a straight line, it depicts a uniform motion $(v=const.,a=0)$. The pitch of the line can then be calculated as $\tan \alpha =\frac{\Delta s}{\Delta t}$.
In the case of constant acceleration $(a=const.)$, the line in a path-time diagram takes the form of a parabola.
位移-
位移-时间图是运动物体的位移s随时间t变化情况的图示。图线的斜率 $\left( \frac{ds}{dt} \right)$ 代表着物体在任意时刻的速度 v。
如果图形是一条直线,它表示物体做匀速运动 $(v=常数,a=0)$。曲线的斜率可计算为 $\tan \alpha =\frac{\Delta s}{\Delta t}$。
在匀加速运动中 $(a=常数)$,位移-时间图中的图线呈抛物线形式。
表示匀度运动的位移-时间图
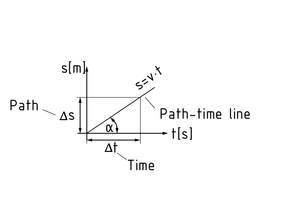
Diagrama ruta-tiempo

Un diagrama ruta-tiempo es una representación gráfica de la ruta s cubierta por un cuerpo en movimiento en el tiempo t. La inclinación $\left( \frac{\Delta s}{\Delta t} \right)$ de la línea correspondiente a la velocidad v del cuerpo en cualquier momento único.
Si la gráfica es una línea recta, representa un movimiento uniforme ( $v=const., a=0$ ). La inclinación de la línea puede entonces ser calculada como $\tan \alpha =\frac{\Delta s}{\Delta t}$ .
En el caso de la aceleración constante $(a=const.)$ , la línea en el diagrama ruta-tiempo toma la forma de una parábola.
Diagrama ruta-tiempo mostrando movimiento uniforme
