Normalbeschleunigung

Komponenten der Radialbeschleunigung

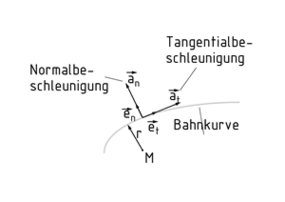
Die Normalbeschleunigung \[{{\vec{a}}_{n}}\] ist eine senkrecht zur Bahnkurve verlaufende Beschleunigung eines Körpers. Die Ursache für eine solch gerichtete Komponente ist das Wirken der Zentrifugal- bzw. Zentripetalkraft.

An einer kreisrunden bzw. gekrümmten Bewegungsbahn ist die Zentripetalbeschleunigung zum Mittelpunkt M des Kreises bzw. des Krümmungskreises gerichtet, während die Zentrifugalkraft die gegensätzliche Orientierung hat.

Tangential zur Bahnkurve verläuft die Tangentialbeschleunigung\[{{\vec{a}}_{t}}\].Sie gibt genau wie der Tangenteneinheitsvektor ${{\vec{e}}_{t}}$ an jeder Stelle der Bahnkurve die Bewegungsrichtung an. Mathematisch ist der Tangenteneinheitsvektor ${{\vec{e}}_{t}}$ die Ableitung der Bahnkurve über die Zeit. Dessen Ableitung wiederum ergibt die Krümmung der Bahnkurve. Zusammengefasst werden die Normal- und Tangentialbeschleunigung als Radialbeschleunigung bezeichnet.

Normal component of acceleration

Components of radial acceleration

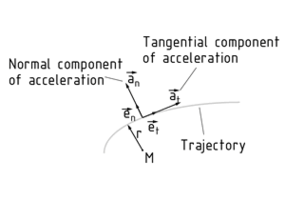
The normal component of acceleration \[{{\vec{a}}_{n}}\] is the Acceleration of a body perpendicular to its trajectory. The cause of this component is the effect of centrifugal and/or centripetal Force.

On a circular or curved trajectory, centripetal acceleration is directed toward the centre point M of the circle or Circle of curvature while centrifugal force has the opposite orientation.

The tangential component of acceleration \[{{\vec{a}}_{t}}\] follows the tangent of the trajectory. It states the direction of movement at each point on the trajectory, just like the unit tangent vector ${{\vec{e}}_{t}}$. In mathematical terms, the unit tangent vector ${{\vec{e}}_{t}}$ is the derivation of the trajectory over time. In turn, the derivation of this reveals the curvature of the trajectory. The normal and tangential components of acceleration are referred to collectively as radial acceleration.

法向加速度

径向加速度分量

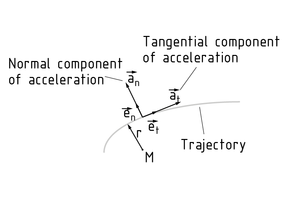
法向加速度 ${{\vec{a}}_{n}}$ 是指垂直于物体运动轨迹方向的加速度。法向加速度是在离心力或向心力或二者共同作用下产生的。

当物体做圆周运动或曲线运动时，向心加速度指向圆心M或曲率圆，当受到离心力的作用时，加速度指向相反方向。

切向加速度 ${{\vec{a}}_{t}}$ 指向轨迹切线的方向。它反映了轨迹上每一点的运动方向，就像单位切向量 ${{\vec{e}}_{t}}$ 。在数学术语中，单位切向量 ${{\vec{e}}_{t}}$ 是指运动轨迹对时间的导数。反过来轨迹的导数也可以表示轨迹的曲率大小。法向加速度和切向加速度统称为径向加速度

径向加速度分量

Componente normal de aceleración

Componentes de la aceleración radial

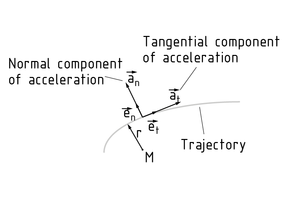
El componente normal de aceleración ${{\vec{a}}_{n}} $ es la aceleración de un cuerpo en dirección perpendicular a su trayectoria. La causa de este componente es el efecto de la fuerza centrífuga o centrípeta.

En una trayectoria circular o curva, la aceleración centrípeta se dirige hacia el punto central M del círculo o el círculo de curvatura, mientras que la fuerza centrífuga tiene la orientación opuesta.

El componente tangencial de la aceleración ${{\vec{a}}_{t}} $ sigue la tangente de la trayectoria. Establece la dirección del movimiento en cada punto de la trayectoria, al igual que el vector de tangente de unidad ${{\vec{e}}_{t}}$ . En términos matemáticos, el vector de tangente de unidad ${{\vec{e}}_{t}}$ es la derivación de la trayectoria en el tiempo. A su vez, la derivación de esto revela la curvatura de la trayectoria. Los componentes normal y tangencial de aceleración son referidos colectivamente como aceleración radial.

Componentes de la aceleración radial